
Princeton University Press, Princeton, 1955. Mathematical Foundations of Quantum Mechanics. Geometry of Quantum Theory, vols I and II. Addison-Wesley, Reading, Mass., Loeve, M. Hrsg, Bharucha-Reid Academic Press, New York, 1970. In fact, a lattice is obtained from a lattice-ordered poset by defining and for any. There is a natural relationship between lattice-ordered sets and lattices. Probabilistic Methods in Applied Mathematics, 53–129. Lattice Theory MathWorld Contributors Insall Lattice-Ordered Set A lattice-ordered set is a poset in which each two-element subset has an infimum, denoted, and a supremum, denoted. Axiomatic Quantum Mechanics and Generalized Probability Theory. Springer- Verlag, Berlin-Heidelberg-New York, 1979.įräser, D.
Uber die begrifflichen Grundlagen der Quantenmechanik Lecture Notes in Physics, 99. Voraussage - Wahrscheinlichkeit - Objekt. Academic Press, New York, 1974.ĭrieschner, M. American Mathematical Society, Providence, R.I.,īirkhoff, G. North-Holland, Amsterdam- London, 1974.īirkhoff, G. University of Queensland, Brisbane, 1973. Grundzüge der theoretischen Logik Springer-Verlag, Berlin-Göttingen-Heidelberg, 1959. Springer-Verlag, New York-Heidelberg-Berlin, 1975. Mathematics Stack Exchange is a question and answer site for people studying math at any level and professionals in related fields. An Algebraic Introduction to Mathematical Logic. Addison-Wesley, Reading, Mass., 1961.īarnes, D. Moderne Anwendungen algebraischer Methoden. Algebraische Betrachtungen zur Theorie des Wirkungsquantums und der Elementarlänge. Applied Boolean Algebra, 2nd ed., Macmillan, New York, 1970. Introduction to Switching and Automata Theory. Walter de Gruyter, Berlin, 1970.įriedell, M. Schaltalgebra und digitale Grundschaltungen. Digitale Elektronik Philips GmbH, Hamburg, 1972.ĭworatschek, S. The greatest lower bound of a, b L is called the meet of a and b and is denoted by a b. Algebra deals with more than computations such as addition or exponentiation. The least upper bound of a, b L is called the join of a and b and is denoted by a b. Discrete Mathematics: Chapter 7, Posets, Lattices, & Boolean Algebra.

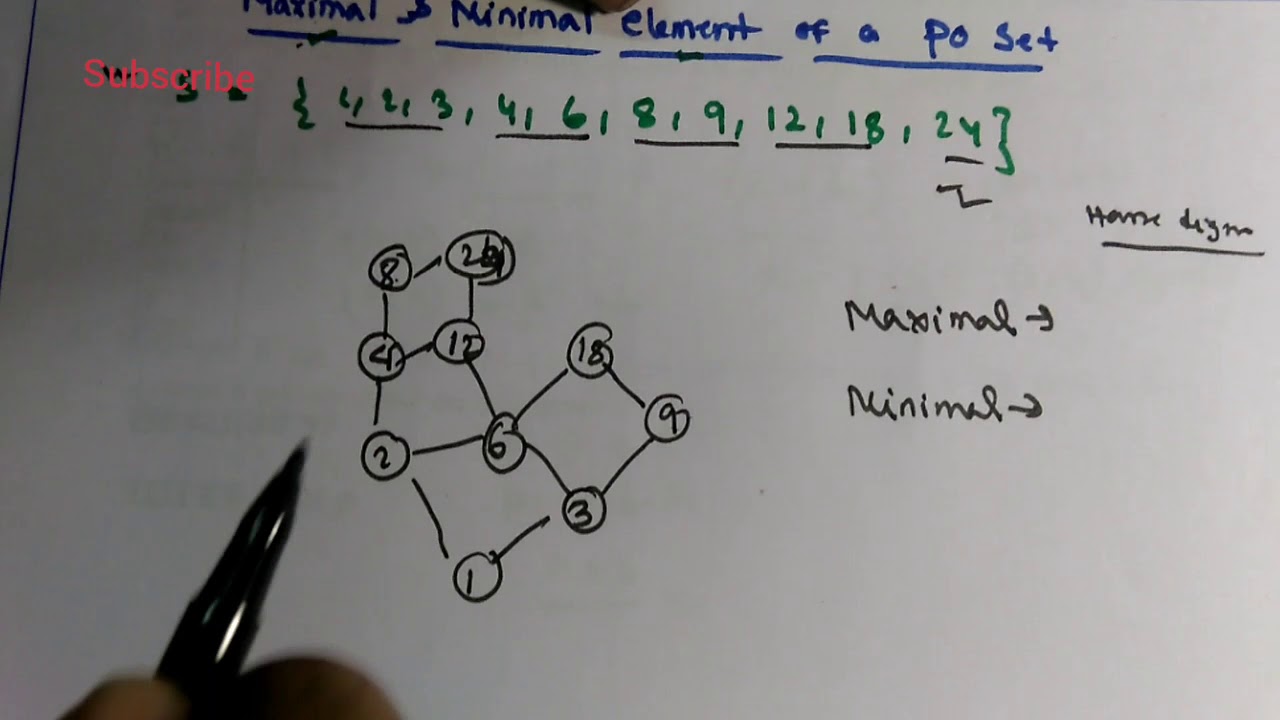
This process is experimental and the keywords may be updated as the learning algorithm improves.ĭokter, F. A lattice is a poset L such that every pair of elements in L has a least upper bound and a greatest lower bound. These keywords were added by machine and not by the authors. The second section considers propositional logic and the third section indicates applications in probability. plumbing systems, road systems with blocks, etc. The same theory will also describe other systems, e.g. It should be noted that the algebra of switching circuits is not described because of its primary importance today but rather for historical reasons since it represented one of the first applications in this field and also because of its elegant mathematical formulation. This application will be described in §1. Ilustrasi Misalkan A sebuah himpunan bilangan bulat positif dan R sebuah relasi biner pada A sedemikian rupa. This is proposition 3.3.1 in Stanley's Enumerative Combinatorics, Volume 1.One of the most important practical applications and also one of the oldest applications of modern algebra, especially lattice theory, is the use of Boolean algebras in modeling and simplifying switching or relay circuits. Definisi Suatu relasi biner dinamakan sebagai suatu relasi pengurutan tak lengkap atau relasi pengurutan parsial (partial ordering relation) jika ia bersifat reflexive, antisymmetric, dan transitive. The meet of two partitions will be their largest common refinement.


 0 kommentar(er)
0 kommentar(er)
